You may use any of these TI calculators:
TI-83 Plus
TI-84 Plus series
TI-Nspire CX series
TI-89 Titanium
TI-73 Explorer
Notable Notes
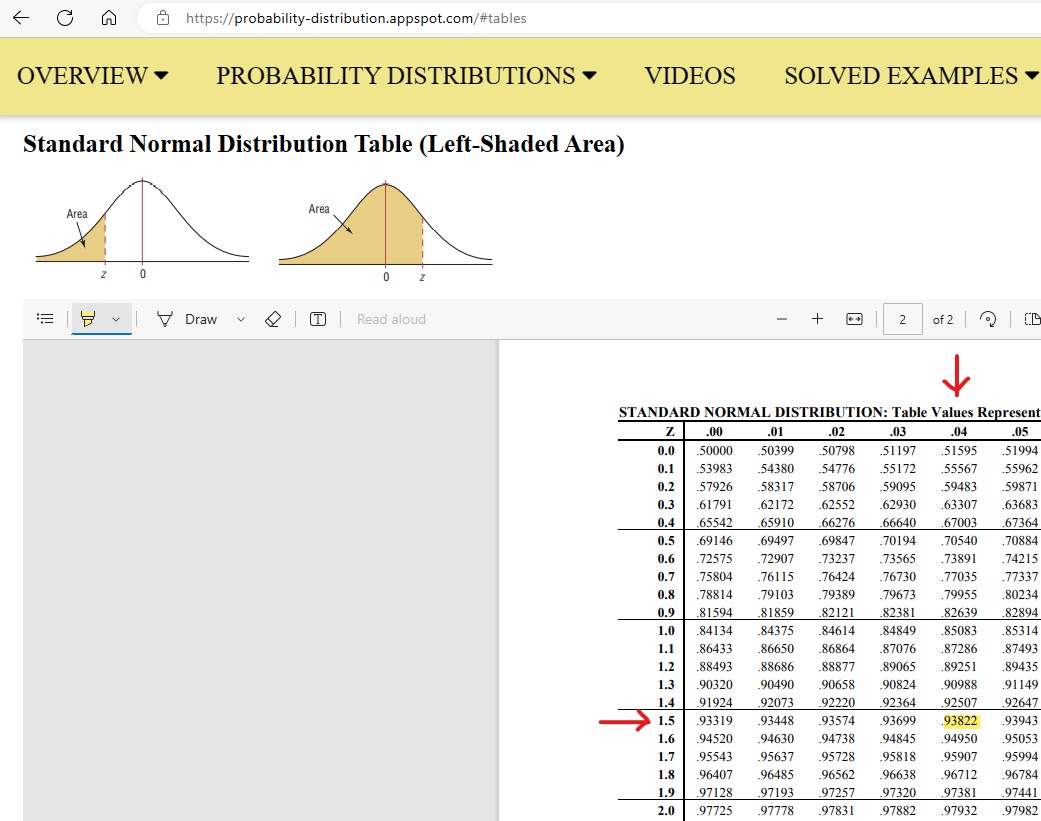
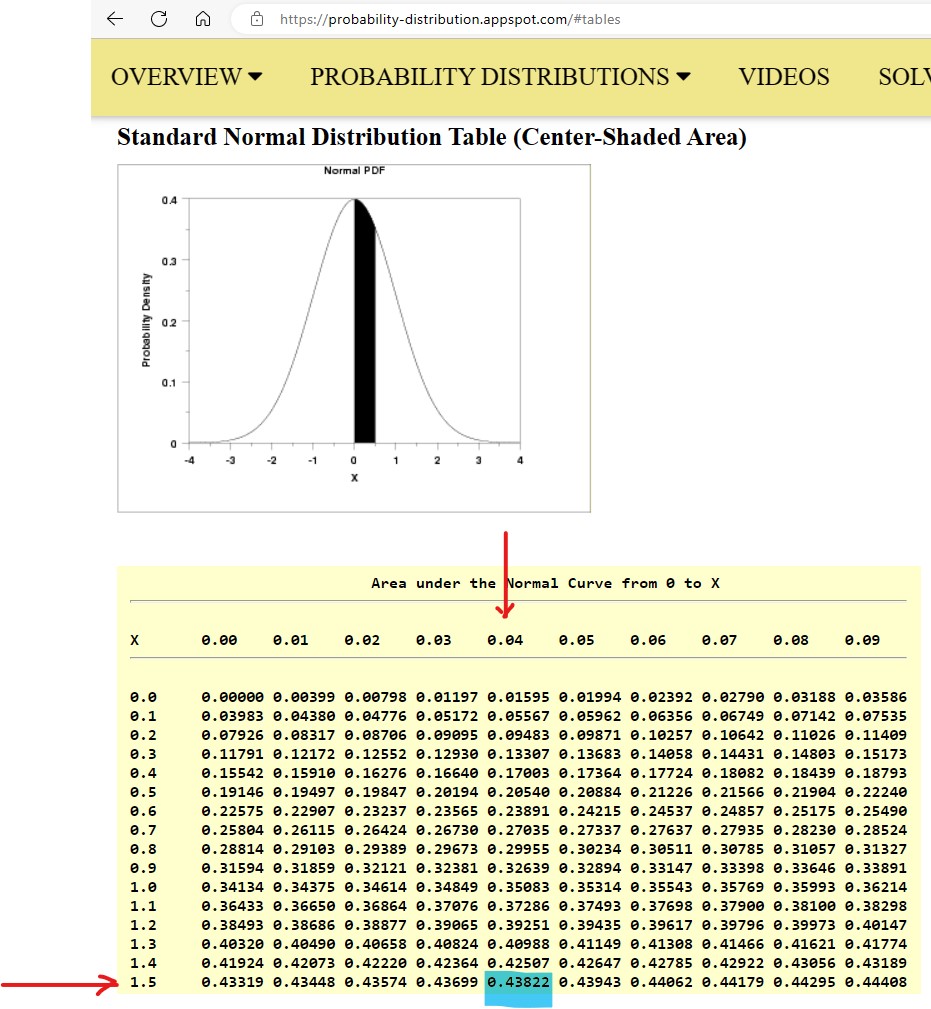
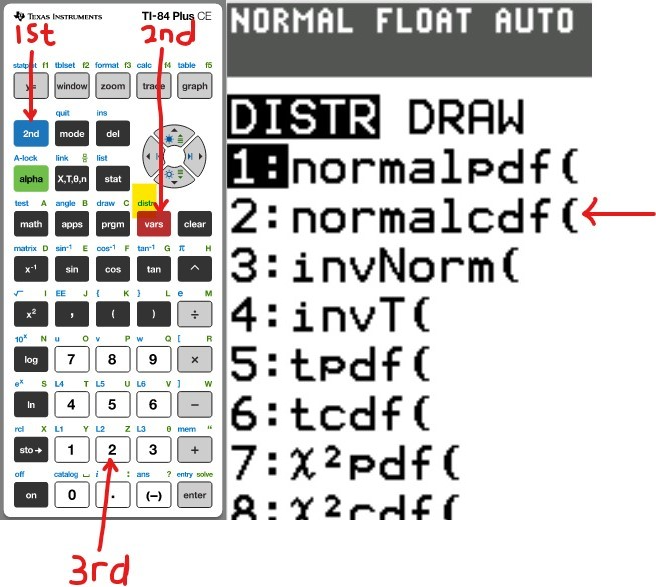
(1.) To find the probability of any probability distribution, use the distribution function DISTR
Because this is in blue color, it can be assessed after 2ND function button is pressed (because the 2ND function button is blue)

(2.) For Normal Distribution:
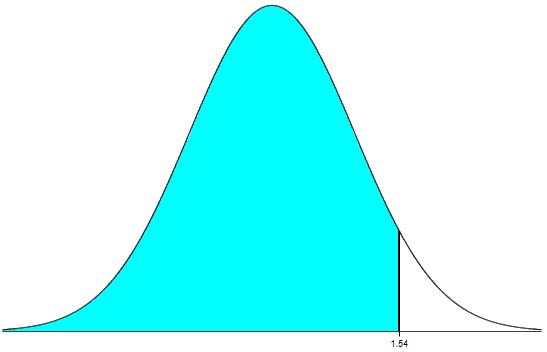
(a.) To find the area or percentage or probability: (i.) less than or equal to a certain value (at most the certain value)
(ii.) more than or equal to a certain value (at least the certain value)
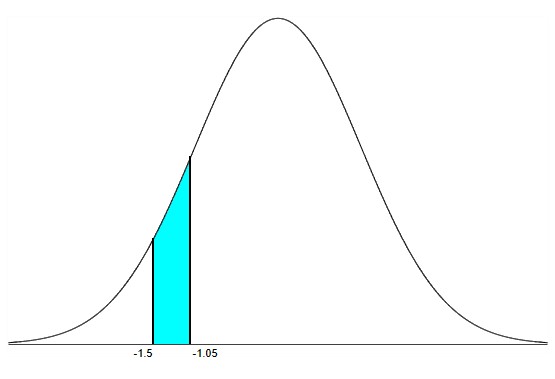
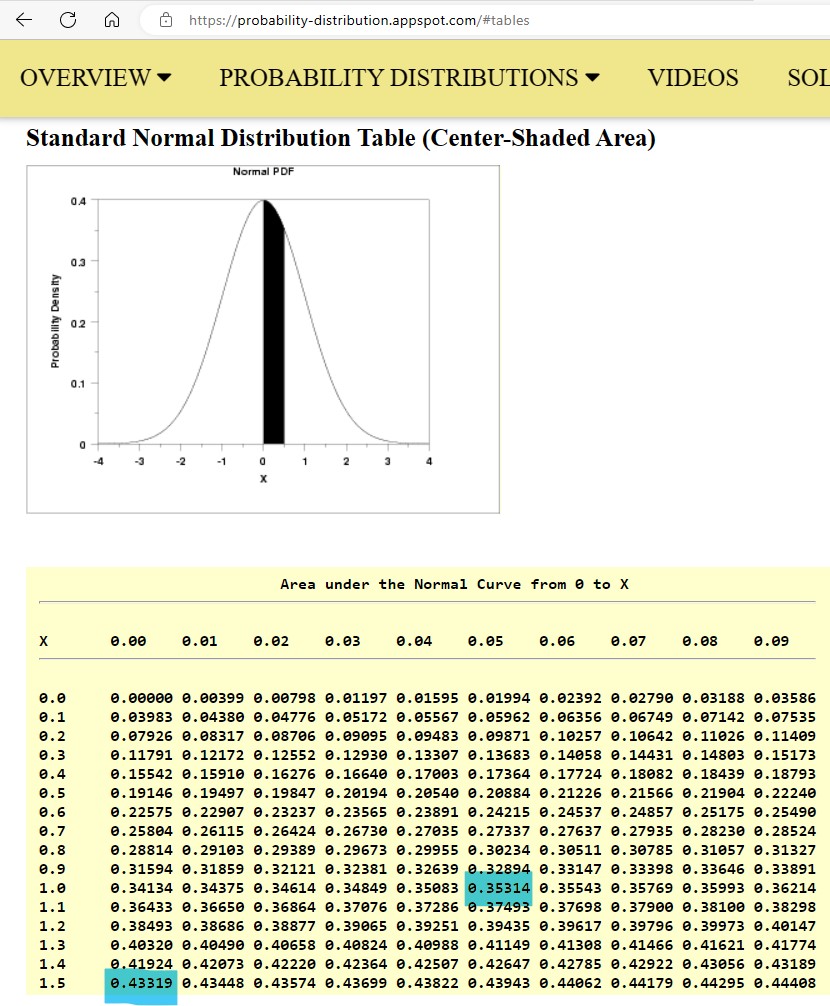
(iii.) between two values
(iv.) away from two values
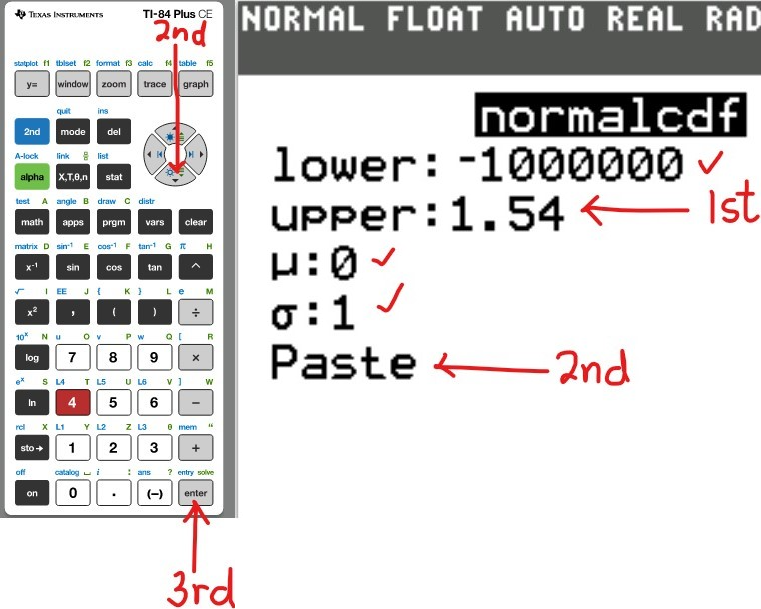
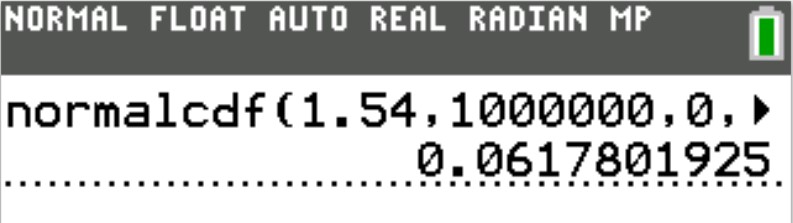
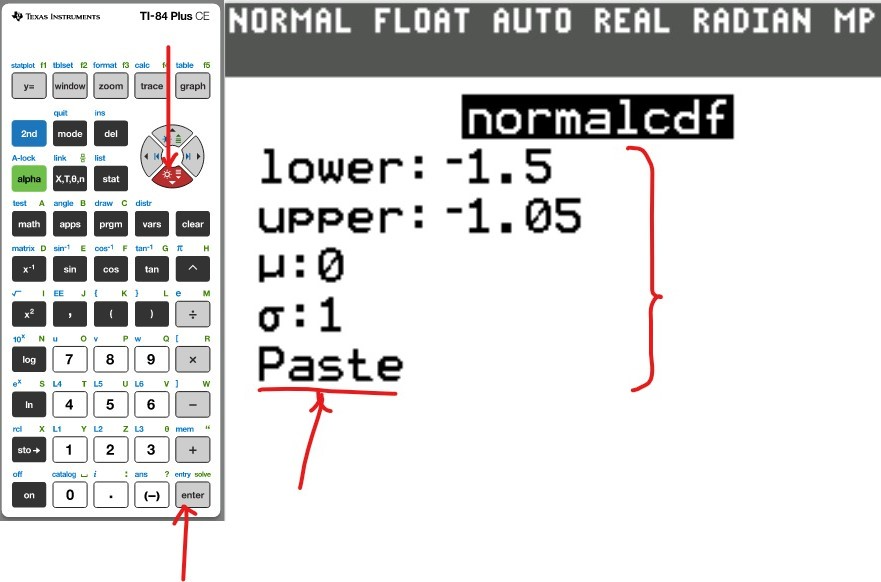
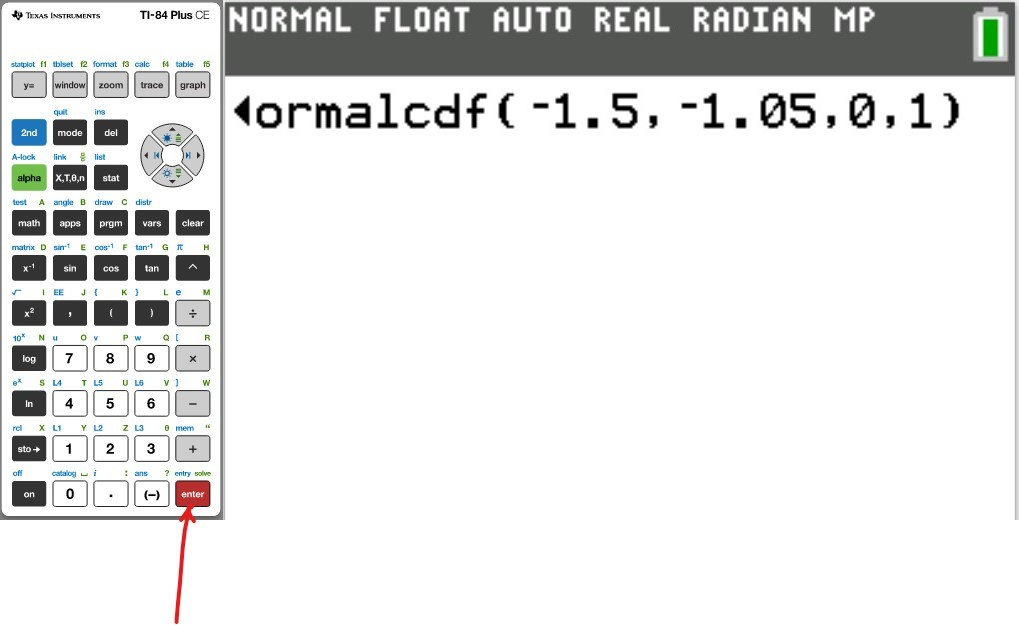
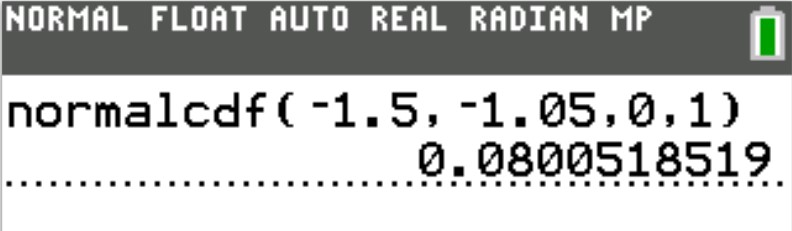
Use the normalcdf (Normal Cumulative Distribution) function
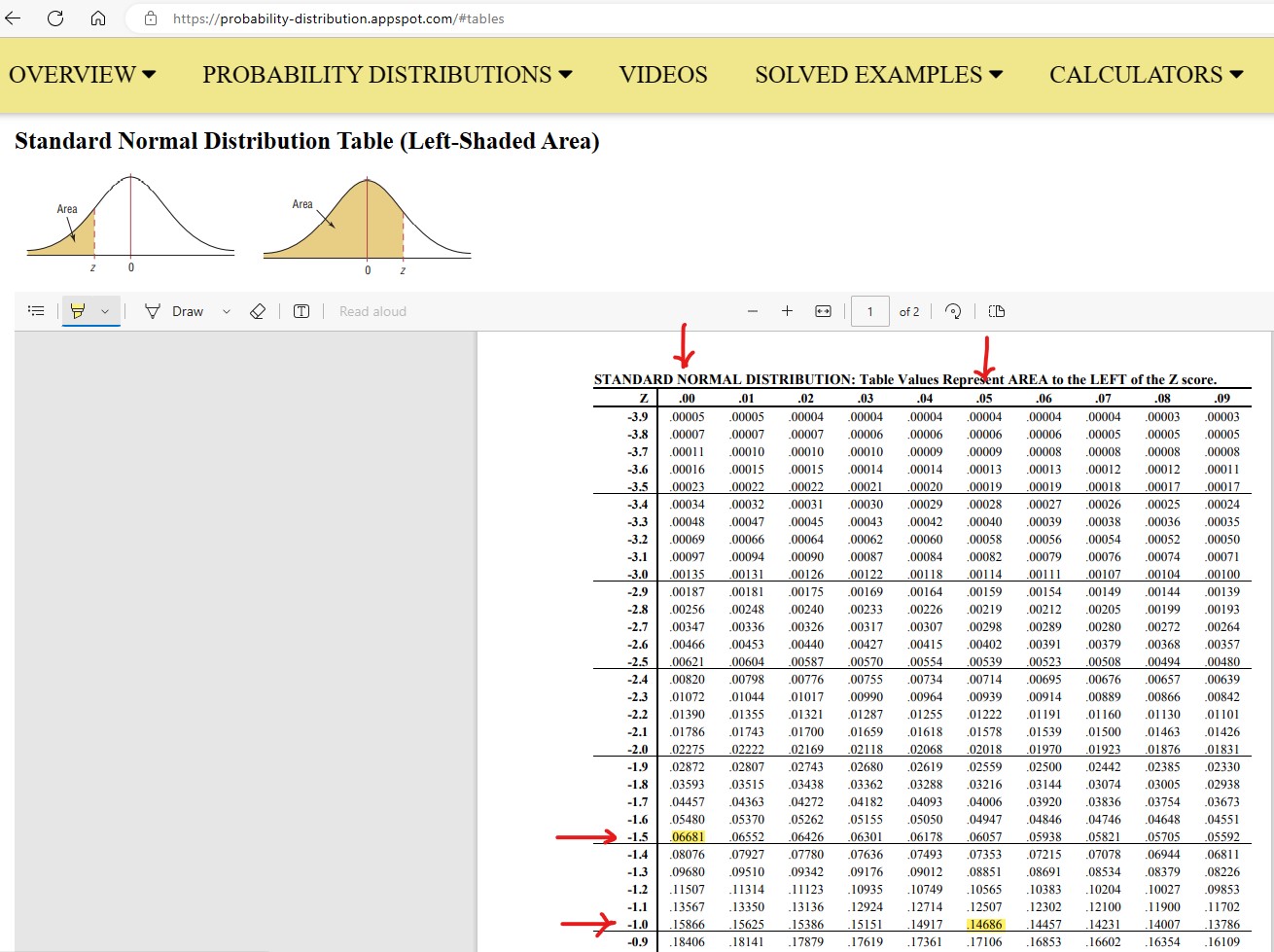
(b.) To find the area or percentage or probability: less than or equal to a certain value (at most the certain value):
(i.) set the lower: −1000000
(ii.) set the upper: certain value

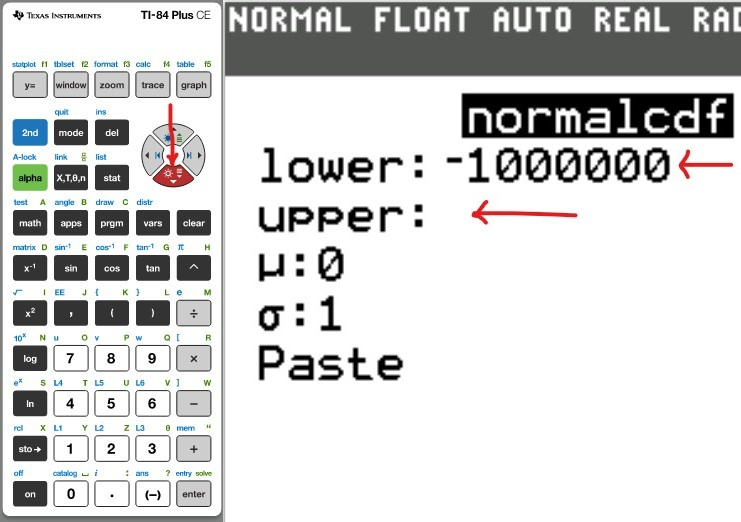
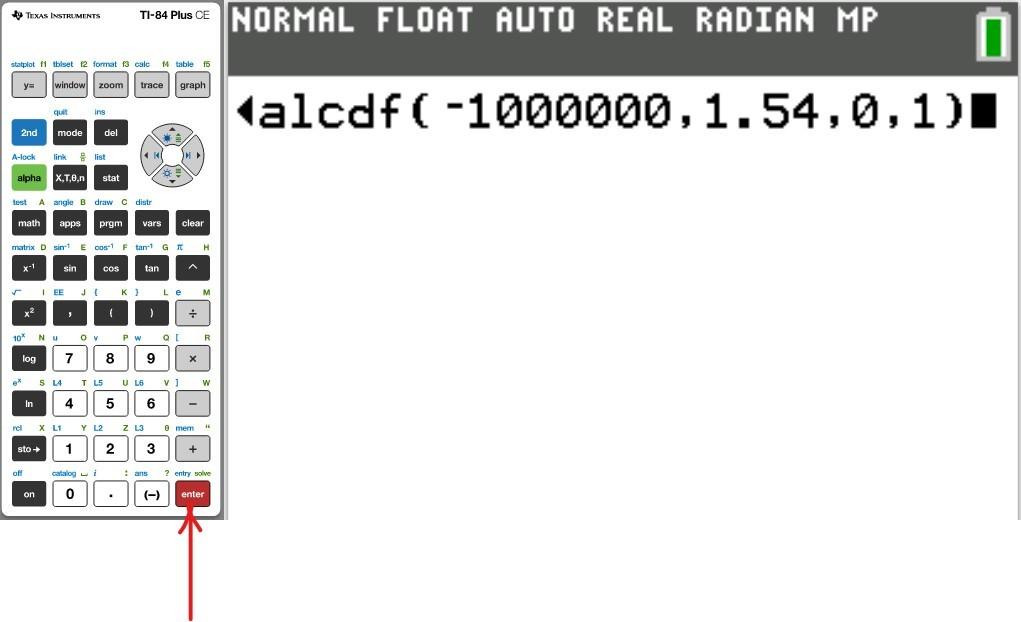
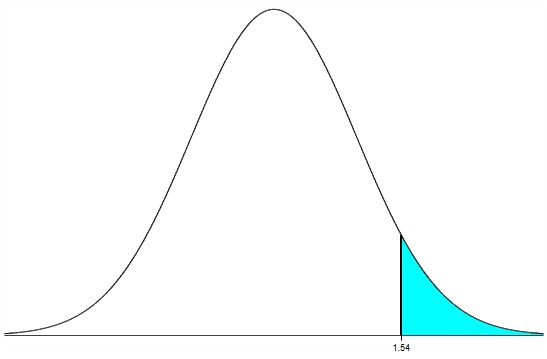
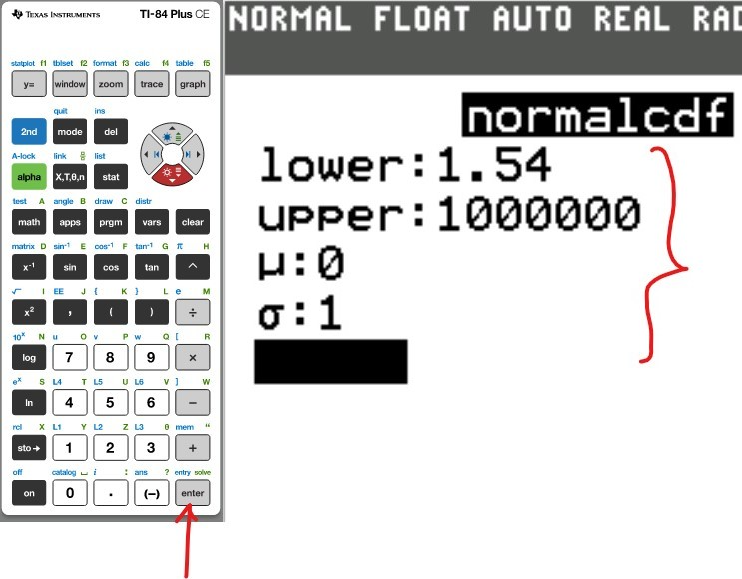
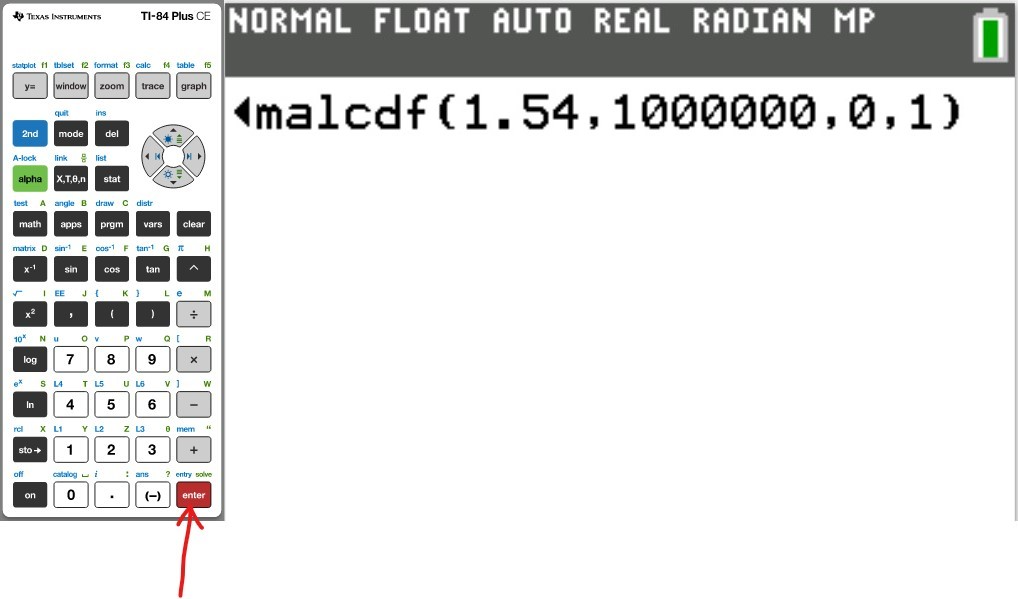
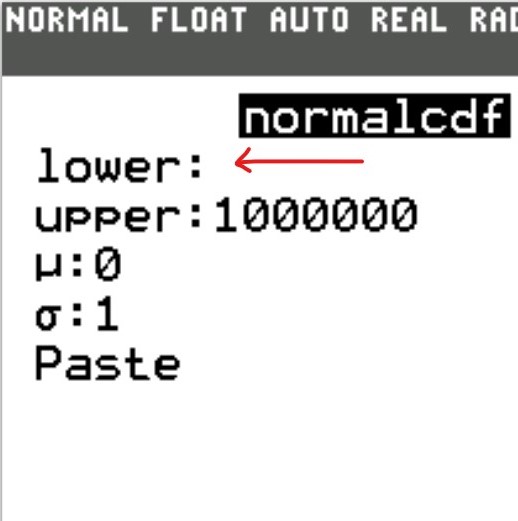
(c.) To find the area or percentage or probability: greater than or equal to a certain value (at least the certain value):
(i.) set the lower: certain value
(ii.) set the upper: 1000000
(d.) To find the area or percentage or probability between two values:
(i.) set the lower: first value
(ii.) set the upper: second value
(e.) To find the area or percentage or probability away from two values:
(i.) set the lower: −1000000
(ii.) set the upper: first value
Added to
(iii.) set the lower: second value
(iv.) set the upper: 1000000
(3.) To find the inverse of any probability distribution, use the distribution function DISTR
Because this is in blue color, it can be assessed after 2ND function button is pressed (because the 2ND function button is blue)
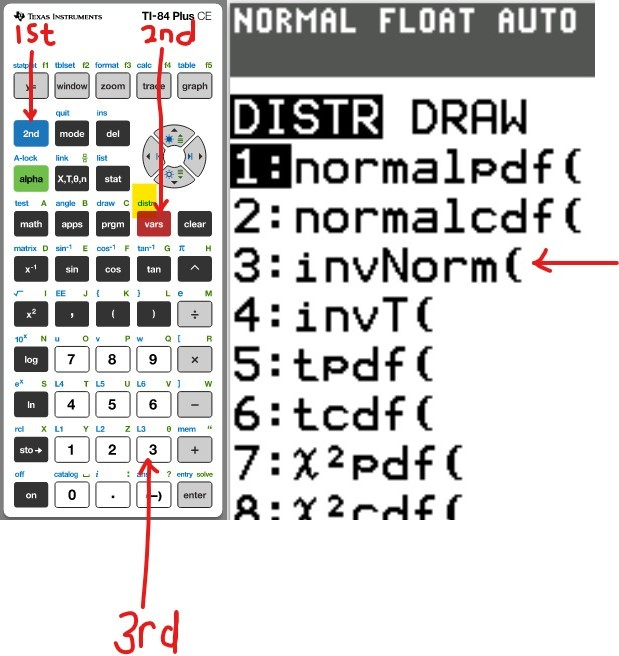
(4.)
Let us do some examples.
NOTE: Please begin from the first example. Do not skip.